After searching far and wide to find a teacher's vehicle new enough to include a back-up sensor system, I then found two "willing" students to help me stage and record an example of the alarm system at work, with visible warning lights above the rear window in the photo shown).

We -- er, actually, I -- backed the vehicle towards our volunteer (pictured here, seen through the vehicle's back window, exercising every ounce of trust she can muster up) and measured her distance to the sensor when it changed intensity and/or volume. The video was simple enough to capture, so I uploaded it to YouTube HERE, if you feel the need to use it in 3-Act-Math format (and because I always enjoy working a Dan Meyer shout-out into a blog post).  My intent was to use the video clip as an Act 1, then the photo below for Act 2 to include some accompanying details and measurements. I have not managed, at this time, to accumulate the necessary camera shots for an Act 3, but hope I can do so in the not-too-distant future.

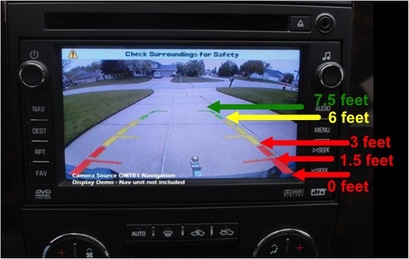I found a screenshot of the new backup sensor/camera system available for the new Chevy Silverado and labeled its colored guidance lines to correspond with the observed distances when the audible and visible alarm system was set off during our trials.

The prompt I gave students was that they needed to be able to provide enough information about the inequalities associated with each tone, so that we could construct a "poor-man's vehicle backing system." To be fair, earlier in the week, we had created several of our own programs to use with the TI-84 Plus (now available in the TI-84 Plus C with color screen and rechargeable battery, which I've grown to appreciate quickly) calculators, including Quadratic Formula, Distance Formula, Slope Formula, and Midpoint Formula programs. I aim to include links to these files as soon as my school webpage editor is properly troubleshot. For now, you may browse my classroom webpage using THIS link.

While this particular prompt may not fit the ideals for the Common Core State Standards for Mathematics, I feel it does give some worthwhile insight into how computer programming logic has a place in a vital component of vehicle safety systems. Sometimes, this sort of insight is enough to squelch the "But when am I ever going to USE THIS STUFF in real life?" questioning that can occur frequently with math topics.

The relationship I had hoped students would construct, or at least mimic, in their analysis of the video clip I created and the accompanying photo shown above.

Most importantly, I wanted to see that students had the wherewithal (yes, I used "wherewithal" in context on a math blog entry) to only include, for example, "6 feet" as part of a single interval instead of assigning it to more than one interval. My hopes in this was to have some sort of reinforcing example to refer to when we begin our look at functions and how each input value can only have one output value.

With this sort of application to the simple, compound, and absolute value linear inequalities we have used this unit, I hope that my students have some sort of foundation or respect for how this topic is relevant and useful in real life. 

The female student in the video seems pretty bold to stand firm, knowing that I'm backing up straight towards her and, truth be told, there was a Counselor's meeting going on while we were filming in the conference room directly behind her. So, I had some explaining to do when I ran across a couple of those counselors towards the end of the school day, but did get some head nodding and encouragement that the lesson potential for what I was doing sounded pretty interesting to them.

In conclusion, this lesson has been the most fun I've had ALMOST intentionally backing over a student in my teaching career. Just wanted to put that out there. Please let me know your thoughts on this lesson and check my Twitter feed to see when I've been able to post those TI-84 programs, if you are interested in when I have those posted. Thanks!

--Keltner--

As the summer wound down, I found myself frequenting home improvement stores to cross of those last few items on my to-do list. In that wandering, I came across a Giant Jenga topic on a Home Depot Community blog by employees/associates.

I figured this would be a great way to tie-in at the beginning of the school year to talk about traits of shapes (in this case squares, so that the Jenga tower has no overlapping from one level to the next). Particularly, I wanted them to address the trait of the assembly that the length of each board was supposed to be the width multiplied by the number of boards stacked across the assembly--in this case, 3 x 5.5" since we went three boards across and each measured 5.5" wide. I was able to toss the idea at the woodworking teacher, who was going to be biding his time in class until students had passed their safety quizzes so they could safely operate the machinery in their shop. He agreed that a straightforward, repetitive project like this one would allow his students to use multiple machines (miter saw, table saw, and planer for example).

Before embarking on the project, I made sure to set the scene with my students and pique their interest in creating their own Giant Jenga. They insisted we Google it to see if someone else had already come up with the idea before them. A sweet collection of games came up, but mostly photos, aside from the Home Depot article I'd mentioned above. They noticed it included measurements (i.e. the answer, they thought). I agree with Dan Meyer here: questions like these are most effective when they are un-Google-able.

I insisted we go bigger than the Home Depot site had done; we should use 2" x 6" boards instead. The task in class that day was for them to help me make out my shopping list. Some prompts we had to answer:

• Why did the Home Depot site mention each board being cut to 10.5" long?
• Would that same length apply when using 2" x 6" boards?
• Could we just buy the longest boards available, or would 8-foot, 10-foot, or 12-foot boards help minimize waste?
• How many, and which length, should I purchase? (When I was at the store, I also discovered the unit rate for these boards differed somewhat; namely that the shorter boards were the cheapest for foot-length. I anticipate revisiting this observation in a later lesson.)

The final product is pictured here. Although it is heavy and not "broken in yet" where the pieces slide easily in and out of their slots, students have enjoyed playing it in the couple of opportunities they have had. The other students from the woodworking class have come by to see the final product in action, since they only saw the raw materials and had not seen the final product in action.

I saw recently where Andrew Stadel had posted about an "experienced" Rubik's cube he held onto and a curious student managed to master quickly. While I have a growing collection of Rubik's cubes and similar puzzles (as seen on the banner for this site, atop the shelves in my classroom with Hoberman spheres and other math-y relics), I hope this Giant Jenga game at least makes an impact on these students since they are the ones who came up with the recipe--or the shopping list, I suppose.

--Keltner--

I feel obligated to include some sort of reference to "The Wizard of Oz," being that I have lived in Kansas my entire life. I try to take advantage of my students' familiarity with the film in several instances though. 

One of my favorite such examples is when the Scarecrow inaccurately recites the Pythagorean Theorem once a brain is decreed upon him (note also how Scarecrow magically moves from the middle of the characters to the far right of the frame after confronting the Wizard). His mishap is recreated by a glasses-wearing Homer Simpson who makes the same mistake, but is corrected by a gentleman in the bathroom stall behind him.

Another mention of "The Wizard of Oz" in class comes up when I emphasize to my students how absolute value measures the distance a number is from zero on a number line. We cannot have a negative distance, so an absolute value will always be non-negative (when a student usually tries to correct me and say "You mean greater than or equal to zero" and suddenly finds that the statements are equivalent).

I paraphrase what the Scarecrow said when first meeting Dorothy (pictured at left): "We can go both ways." The same can be said of absolute values, in that they measure the same distance from a central reference point; in the general case, a number's distance from zero.

In introducing the concept of absolute value this year in Algebra 2, I emphasized greatly the importance of that feature, that absolute value equations would measure the same distance from a central reference point. Refer to the example here:

Solve the equation | x - 2 | = 3. Check your solutions in the original equation.

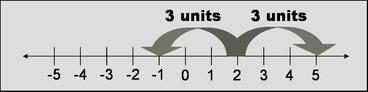I interpreted the equation, telling them that it is asking, in Algebra terms, "What number's distance from 2 is 3 units?"

They started talking, but only focusing on using that distance in the positive direction, citing 5 as the only solution. This diagram helped them clarify that -1 is also 3 units away from 2. We substituted each value back into the original equation to check our work and conclude that each apparent solution was indeed a valid solution (a great time to introduce the idea of extraneous solutions and emphasize how our conclusion would have differed if we were discussing money, volume, unit sales, and other applications.

I then decided to give an open-ended task to end class that day. I asked students to find something in real life around them that involved the same distance away from a central point. The example I gave was that I parked my car that morning so it was the same distance away from the striped lines on each side of my parking spot, making sure to point out how disappointed I would be if all students returned the next day having simply taken a photo of their car in the parking lot. They came through with some great examples, as shown in the slide show below.

Students brought their cell phones to class and were able to display a screen shot using the IPEVO Point2View document camera I have on my desk (they are excellent for what I use it for and allow quick, easy interface via USB interface including quick screen shots when desired). They were able to sketch on top of their photos using the SMART Board in my room as well, even being able to accept suggestions from classmates on examples of absolute value within their own photo that they had not acknowledge.

I aim to do more of these sort of open-ended, scavenger hunt-like examples where students find examples of math around them. I'll call it "Have My Stu's Do A 101Q" to give proper credit to Dan Meyer's 101qs.com website for posting examples of photos meant to drive student curiosity. I might even be able to coerce some of them into posting to Twitter with a class-named hashtag.  Only time will tell. Like the Scarecrow said, it "could go both ways."

--Keltner--

I become curious at the layout of clocks used in classrooms from time to time. Some clocks digital, some analog. Those with 24-hour time format "cheat sheets" like the one at left bring up a curious topic in math I do not often get to incorporate directly into regular course curriculum, but I think it bears mentioning. 

Allow me to dwell on the 24-hour time format a bit longer, with a military focus. Here are some phrases commonly heard (or slightly paraphrased) in movies associated with the military:

"Reveille is at 0500." Fine. I understand that. They blow the bugle at 5 a.m.

"Lunch at 1200." Gotcha, I know that means at noon. Just tell me where.

"Maverick, there's a bogey on your SIX." No need for an explanation. Hit the brakes and watch him fly by. If only I had a nickel each time I made a "Top Gun" reference with my students and colleagues...

But "Debriefing at 1630"!?!? I have to pause and ponder for a little bit. 

My mind thinks "How many hours past 1200 is that?" Therein lies an example of the importance of remainders.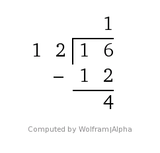

Military time, or 24-hour time, is a very useful and visible example of why remainders play an important role in everyday life. This is one topic I find interesting to emphasize among my students when the opportunity presents itself. 

Whether students/soldiers/civilians/passersby realize or not, they have lunged headlong into a key concept in number theory: modular arithmetic.

I created (what I felt at the time was) a good introductory worksheet on modular arithmetic, using students' complaints about "always having the same thing for lunch" and made up a rotating set of dishes served in a neighboring school's cafeteria. A scan of the original, older version of it and the answer key is available HERE, if interested.

Symbolically, this tells us that 16 == 4(mod 12), or that when divided by 12, the numbers 16 and 4 have the same remainder.  By similar measure, I could quickly determine that a football coach saying "kickoff is at 1900" is not just due to the fact he is a social studies teacher and currently talking about the 1900's in U.S. History class.  Since 19 == 7(mod 12), he is referring to 7:00 p.m. for kickoff time.

But remainders go beyond just telling time. Suppose today is the 5th and I need to know what day of the week the 19th falls on? You may not use the word "remainder" but somewhere in your logic, the concept does rear its head at you. Admit it.

Remainders become prevalent in other, more visible ways, around us in everyday life.  Try these scenarios:

• You are in line at the store and an item's bar code does not scan, so the clerk enters the UPC manually, only to receive an "Invalid UPC" prompt on their register.
• You need to order a textbook for a graduate course, but find the book title, subtitle, edition, author(s), publisher, and publish date to be an exhaustive list of information to compile to verify you order the appropriate book.
• You are baffled by the small series of tick marks that appear on junk mail you receive, but have a hunch it helps automatic sorters at the Post Office begrudgingly deliver those items in a quick, efficient manner without having to read your misspelled name and incorrect address or, my favorite, "Or Current Resident" on the name line.

These are all valid applications of UPCs, ISBNs, and IMBs; and no, that's not a competition for more acronyms than you'll hear in a staff meeting about NCLB, CCSS, or SBG. 

I tend to focus here on UPCs and ISBNs, because they are the most readily available examples I use in class when a student brings up this topic.  Not once have I been interrupted with a "Do we need to know this for the test?" I like to think it is because students are engaged in the topic, since it is applicable to a plethora of things around them.